Question

Darlene is adopting a pet. she can choose from 8 kittens and 6 puppies.How many ways can she choose a pet to adopt

Answer 1

14 cause shes only getting 1 pet and there are 14 animals in total

Answer 2

Answer: The required number of ways is 14.

Step-by-step explanation: Given that Darlene is adopting a pet and she can choose from 8 kittens and 6 puppies.

We are to find the number of ways in which she can choose a pet to adopt.

We have

the total number of pets that are available to choose from is given by

`n=8+6\\\\\Rightarrow n=14.`

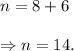
Since Darlene is going to adopt only one pet, so we have

`r=1.`

Therefore, the number of ways in which she can choose a pet to adopt is given by

`^nC_r\\\\=^(14)C_1\\\\\\=(14!)/(1!(14-1)!)\\\\\\=(14!)/(1!13!)\\\\\\=(14*13!)/(1*13!)\\\\=14.`

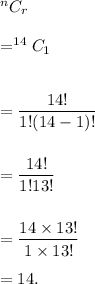
Thus, the required number of ways is 14.