Question

In slope-intercept form, what is the equation of the line passing through the points (−4, 26) and (6, −4)?

y = −3x − 14
y = 3x + 14
y = −3x + 14
y = −3x + 2

Answer 1

y=-3x+14 is the answer

Answer 2

Option C is correct.

Explanation:

Given: Passing through Point are ( -4 , 26 ) and ( 6 , -4 )

We have to write the equation of the line passing through given point in Slope-Intercept Form.

First we find the equation using Two-point form then rewrite in slope-intercept form.

Two Point Form,

`y-y_1=(y_2-y_1)/(x_2-x_1)(x-x_1)`

`y-26=(-4-26)/(6-(-4))(x-(-4))`

`y-26=(-4-26)/(6+4)(x+4)`

`y-26=(-30)/(10)(x+4)`

`y-26=-3(x+4)`

`y=-3x-12+26`

`y=-3x+14`

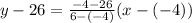
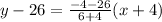
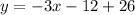
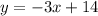
Therefore, Option C is correct.