Answer:
The value of
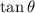 is
is
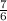 .
.
Explanation:
It is given that a straight line inserted at the origin terminates at the point (6, 7) as it sweeps out an angle θ in standard position.
Draw a diagram using the given information.
From the given graph it is clear that it is a right angled triangle with base 6 and height 7.
According to the trigonometric ratios,
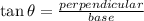
Substitute perpendicular=7 and base=6 in the above equation.

Therefore the value of
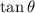 is
is
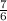 .
.