Question

 A train covered the distance of 400 km between A and B at a certain speed. On the way back it covered 2/5 of the distance at that same speed and then it decreased its speed by 20 km/hour. Find the speed of the train at the end of its journey from B back to A, if the entire trip took 11 hours.

Answer 1

60 km

Explanation:

Answer 2

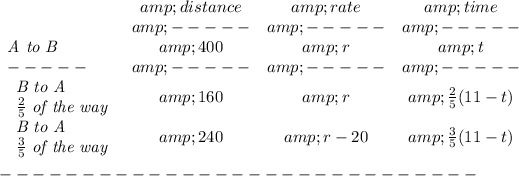
so hmmm the train goes one way, from A to B, 400 km, takes hmm say "t" time, it goes at rate "r"

then it comes back around, at rate "r", goes 2/5 of the way, then it drops its speed to "r - 20", or 20km less per hour

that means, the rest of the way on the B to A way, it's only running at r-20, now, a "whole" is 1, if we take 2/5 then we're left with 3/5, so 3/5 of the way from B to A, it went r-20 fast

since the whole trip, A to B and B to A, took 11hrs total, then if from A to B, it took "t" time, from B to A, it took 11-t long

2/5 of 400 is 160km, and 2/5 of 11-t is 2/5(11-t)
now
3/5 of 400 is well, 240 :), and 3/5 of 11-t is 3/5(11-t)

if we add 160 + 240, is 400, since 2/5 + 3/5 is 5/5 or 1whole

so, if we add 2/5(11-t) + 3/5(11-t), is how long it took on the way from B to A

so.. we that in mind


`\bf \begin{array}{lccclll} &distance&rate&time\\ &-----&-----&-----\\ \textit{A to B}&400&r&t\\ -----&-----&-----&-----\\ \begin{array}{llll} \textit{B to A}\\ (2)/(5)\textit{ of the way} \end{array}&160&r&(2)/(5)(11-t)\\ \begin{array}{llll} \textit{B to A}\\ (3)/(5)\textit{ of the way} \end{array}&240&r-20&(3)/(5)(11-t) \end{array}\\\\ -----------------------------\\\\`


`\bf \begin{cases} \boxed{400}=rt\implies \cfrac{400}{r}=t\\\\ --------------------------\\\\ 160=r\left[ \cfrac{2}{5}(11-t) \right]\implies 160=r\left[ \cfrac{2}{5}\left(11-\cfrac{400}{r}\right) \right]\\\\ 240=(r-20)\left[ \cfrac{3}{5}(11-t) \right]\implies 240=(r-20)\left[ \cfrac{3}{5}\left(11-\cfrac{400}{r}\right) \right]\\\\ ----------------------------\\\\ 160+240=r\left[ \cfrac{2}{5}\left(11-\cfrac{400}{r}\right) \right]+240=(r-20)\left[ \cfrac{3}{5}\left(11-\cfrac{400}{r}\right) \right] \end{cases}`


`\bf \boxed{400}=400\qquad meaning\\\\ 400=r\left[ \cfrac{2}{5}\left(11-\cfrac{400}{r}\right) \right]+240=(r-20)\left[ \cfrac{3}{5}\left(11-\cfrac{400}{r}\right) \right] \\\\\\ \textit{let us, multiply, both sides by 5, and distribute} \\\\\\ 2000=[22r-800]+\left[ 33r-1860+\cfrac{24000}{r} \right] \\\\\\ 2000=55r-2660+\cfrac{24000}{r}\implies 0=55r-4660+\cfrac{24000}{r} \\\\\\ \textit{let's multiply both sides by`

now, if you run the quadratic formula on that quadratic, you'd get two values, one is a low 5.5 or 5.5 km/h, which is very unlikely the train was going that fast =), and you'd get a feasible 79.2189, or about 79km/h