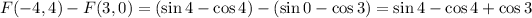You can parameterize the curve
and compute the integral along each component curve, or you can use the fact that
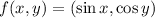
is the continuous gradient of a function
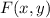
and observe that the line integral is path independent.
In other words, there is a function
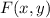
such that
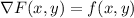
, so the integral along any curve
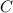
from the points
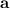
to
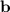
is simply
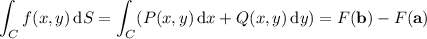
You have
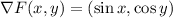
, and so

while
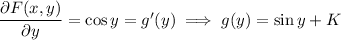
where

is an arbitrary constant. So we've found that
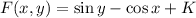
which means the line integral has a value of