Question

Construct a polynomial function with the stated properties. Reduce all fractions to lowest terms.Third-degree, with zeros of -2, - 1, and 3, and passes through the point (2, 7).

Answer 1

`f(x)=-(7)/(12)x^3+(49)/(12)x+(7)/(2)`

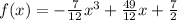
Step-by-step explanation

If x1, x2, x3, ..., xn are the zeros of a polynomial P, then the polynomial can be written as the product of the factors,

`P(x)=(x-x_1)(x-x_2)(x-x_3)\ldots(x-x_n)`

In this problem we have a third-degree polynomial function, so it has 3 zeros and thus 3 factors,

`f(x)=a(x+2)(x+1)(x-3)`

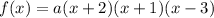
We have to find a knowing that the function has to pass through point (2, 7). This means that when x = 2, f = 7,

`f(2)=7`

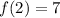
Replace into the function,

`7=a(2+2)(2+1)(2-3)`

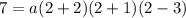
Solve the parenthesis,

`7=a(4)(3)(-1)`

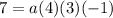
Multiply,

`7=a(-12)`

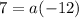
And solve for a by dividing both sides by -12,

`\begin{gathered} (7)/(-12)=(a(-12))/(-12) \\ a=-(7)/(12) \end{gathered}`

Hence, the function is

`f(x)=-(7)/(12)(x+2)(x+1)(x-3)`

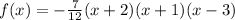
Next, we have to multiply the factors to obtain the function in standard form. Multiply the first two,

`f(x)=-(7)/(12)(x\cdot x+2x+1x+2\cdot1)(x-3)`
`f(x)=-(7)/(12)(x^2+3x+2)(x-3)`

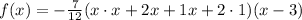
Then multiply by the last factor,

`f(x)=-(7)/(12)(x^2\cdot x+3x\cdot x+2\cdot x-3\cdot x^2-3\cdot3x-3\cdot2)`
`f(x)=-(7)/(12)(x^3+3x^2+2x-3x^2-9x-6)`

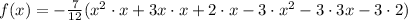
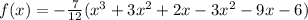
Add like terms,

`f(x)=-(7)/(12)\lbrack x^3+(3x^2-3x^2)+(2x-9x)-6\rbrack`
`f(x)=-(7)/(12)(x^3-7x-6)`

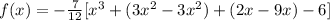
And finally, distribute the coefficient,

`f(x)=-(7)/(12)x^3+(7)/(12)7x+(7)/(12)6`
`f(x)=-(7)/(12)x^3+(49)/(12)x+(7)/(2)`

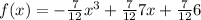
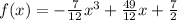
This is the polynomial function with zeros -2, -1 and 3 that passes through point (2, 7)