Answer:
see explanation
Explanation:
Using the chain rule
Given
y = f(g(x)), then
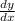 = f'(g(x)) × g'(x) ← chain rule
= f'(g(x)) × g'(x) ← chain rule
and the standard derivatives
 (
(
 x ) =
x ) =
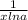 ,
,
 (lnx) =
(lnx) =
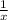
(a)
Given
y =

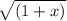
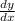 =
=
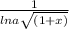 ×
×
 (
(
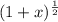
=
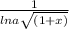 ×
×

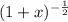 ×
×
 (1 + x)
(1 + x)
=
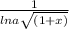 ×
×
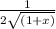 × 1
× 1
=
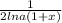
=

(b)
Given
y = ln sinx
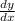 =
=
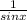 ×
×
 (sinx)
(sinx)
=
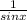 × cosx
× cosx
=
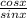
= cotx