Question

The price that a company charged for a basketball hoop is given by the equation 50-5x^2 where x is the number of hoops that are produced, in millions. It costs the company $30 to make each basketball hoop. The company recently reduced its production to 1 million hoops but maintained its profit of 15 million dollars. Approximately how many basketball hoops did the company previously produce to make the same profit?

1.3 million hoops
1.4 million hoops
15 million hoops
30 million hoops

Answer 1

1.3 million hoops did the company previously produce to make the same profit.

Explanation:

Given: An equation for the price of the basket hoop:
`50-5x^2`.

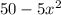The cost that company make each basketball hoop is
`\$ 30`.

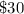Let the number of hoops in million be x.

First determines the profit,

We multiply the given equation with the number of hoops sold and then subtracted by the cost make hoops.

Then, Profit=Total Revenue-Total Cost

Profit=
`\left (50-5x^2 \right)x-30x`

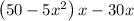
We have to find the number of basketball hoop did the company previously to yield a profit of 15 million dollars.

∴
`\left (50-5x^2 \right)x-30x=15`

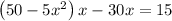
Now, by use of graphical solution:

we get the value of x=1.3 million hoops(Approximately).

Therefore, Approximately 1.3 million basketball hoops did the company previously produce to make the same profit $15 million.