Question

Find the missing side. Round to the nearest tenth. y=?

Answer 1

I believe it’s 140 I rounded it and got 140 so it should work

Answer 2

The length of side
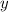`\( y \)` is approximately
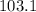`\( 103.1 \)` when rounded to the nearest tenth.

To find the missing side y of the right-angled triangle, we can use the trigonometric functions. Given the angle of
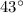`\( 43^\circ \)` and the opposite side x, we can use the tangent function to find side y. The tangent of an angle in a right triangle is the ratio of the opposite side to the adjacent side.

Here's the step-wise method:

1. Set up the equation using the tangent function:

`\[ \tan(43^\circ) = (x)/(y) \]`

2. Since we know the length of the hypotenuse (141), we can use the sine function to find x, and then solve for y using the tangent function. For the sine function:

`\[ \sin(43^\circ) = (x)/(141) \]`

3. Solve for x:

`\[ x = 141 * \sin(43^\circ) \]`

4. Once x is found, rearrange the tangent function to solve for y:

`\[ y = (x)/(\tan(43^\circ)) \]`

5. Plug in the value of x into the equation to find y.

Let's perform the calculations.

To find the missing side y of the right triangle, here are the steps and the calculated values:

1. We set up the equation using the tangent function for angle
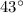`\( 43^\circ \)` and side x:

`\[ \tan(43^\circ) = (x)/(y) \]`

2. We use the sine function to find the length of side x (the side opposite to the
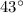`\( 43^\circ \)` angle):

`\[ \sin(43^\circ) = (x)/(141) \]`

3. We calculate the length of side x:

`\[ x = 141 * \sin(43^\circ) \]`

`\[ x \approx 96.2 \]`

4. We rearrange the tangent function to solve for y:

`\[ y = (x)/(\tan(43^\circ)) \]`

5. We plug in the value of
`\( x \)` into the equation to find y:

`\[ y \approx (96.2)/(\tan(43^\circ)) \]`

`\[ y \approx 103.1 \]`

Hence, the answer is approximately
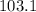`\( 103.1 \)`.