Step-by-step explanation:
• x: number of ,children, that swam at the public pool
,
• y: number of ,adults, that swam at the public pool
We can write a system of linear equations:
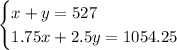
Using the substitution method we can clear y from the first equation:
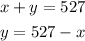
Replace into the second equation:
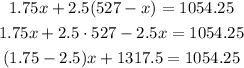
And solving for x:

Replacing x = 351 in the first equation we can find y:
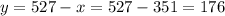
Answer:
• Children: ,351
,
• Adults: ,176