Question

Credit card A offers an introductory APR of 4.1% for the first 3 months and a standard APR of 18.5% thereafter, while credit card B offers an introductory APR of 3.7% for the first 3 months and a standard APR of 18.9% thereafter. All else being equal, which of these statements is correct? (Assume all interest is compounded monthly.)

Answer 1

The correct statement is that credit card B has a lower effective interest rate over the first 3 months compared to credit card A.

Step-by-step explanation:

The correct statement is that credit card B has a lower effective interest rate over the first 3 months compared to credit card A.

To determine the effective interest rate, we can use the formula:

Effective Interest Rate =
`(1 + APR/12)^12 - 1`

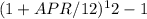
For credit card A, the initial APR is 4.1%, so the effective interest rate over the first 3 months would be -

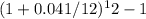`(1 + 0.041/12)^12 - 1` = 0.04203 or 4.203%.

For credit card B, the initial APR is 3.7%, so the effective interest rate over the first 3 months would be -

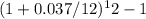`(1 + 0.037/12)^12 - 1` = 0.03728 or 3.728%.

Therefore, credit card B has a lower effective interest rate during the introductory period, making it the correct statement.

Answer 2

Credit card A
First 3 months:
4.1% / 360 = 0.011% x 30 = 0.34% per month for the first 3 months.
Next 9 months:
18.5% / 360 = 0.051% x 30 = 1.54% per month for the next 9 months.

Credit card B:
First 3 months
3.7% / 360 = 0.010% x 30 = 0.30% per month for the first 3 months
Next 9 months:
18.9% / 360 = 0.0525% x 30 = 1.575% per month for the next 9 months

Credit Card B is the better deal for the first 3 months.
Credit Card A is the better deal for the next 9 months.