Apply the Angles Sum Property to the triangle DEF,
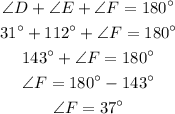
According to the Sine Rule, the length of side is proportional to the angles measure opposite to it. So the shortest side will be opposite to shortest angle, and the largest side will be opposite to the largest angle.
It is observed that,
[tex]DThis follows that,[tex]EFThus, the order of sides from shortest to longest is
EF, DE, DF.