Question

Use coordinate methods (for example, distance formula, midpoint formula, or slope formula) to provethese triangles are congruent.C(-1,2) (-2,0)A(-3,-2) B(4,-3)D23B

Answer 1

To check for the congruency of the triangle, we will attempt to prove the Side-Side-Side (SSS) Congruence Postulate.

This we can do by finding the distance of the line segments of the triangles.

The Distance Formula is given as

`r=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2}`

The coordinates of the vertices are:

`\begin{gathered} A\to(-3,-2) \\ B\to(4,-3) \\ C\to(-1,2) \\ D\to(-2,0) \end{gathered}`

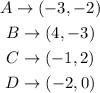
Length of AB:

`\begin{gathered} AB=\sqrt[]{(4-\lbrack-3\rbrack)^2+(-3-\lbrack-2\rbrack)^2} \\ AB=\sqrt[]{7^2+\lbrack-1\rbrack^2}=\sqrt[]{49+1} \\ AB=\sqrt[]{50} \end{gathered}`

Length of BC:

`\begin{gathered} BC=\sqrt[]{(-1-4)^2+(2-\lbrack-3\rbrack)^2} \\ BC=\sqrt[]{\lbrack-5\rbrack^2+5^2}=\sqrt[]{25+25} \\ BC=\sqrt[]{50} \end{gathered}`

Length of AD:

`\begin{gathered} AD=\sqrt[]{(-2-\lbrack-3\rbrack)^2+(0-\lbrack-2\rbrack)^2} \\ AD=\sqrt[]{1^2+2^2}=\sqrt[]{1+4} \\ AD=\sqrt[]{5} \end{gathered}`

Length of CD:

`\begin{gathered} CD=\sqrt[]{(-2-\lbrack-1\rbrack)^2+(0-2)^2} \\ CD=\sqrt[]{\lbrack-1\rbrack^2+\lbrack-2\rbrack^2}=\sqrt[]{1+4} \\ CD=\sqrt[]{5} \end{gathered}`

For the SSS Congruence Postulate to apply, we must have 3 congruent sides.

From our calculations above, we have

`\begin{gathered} |AB|\equiv|BC| \\ |AD|\equiv|CD| \end{gathered}`

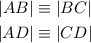
Note that |DB| is a common side in both triangles.

This proves that both triangles are congruent.