Question

A thin nonconducting rod with a uniform distribution of positive charge Q is bent into a circle of radius R. The central perpendicular axis through the ring is a z-axis, with the origin at the center of the ring.

(a) What is the magnitude of the electric field due to the rod at z = 0? N/C

(b) What is the magnitude of the electric field due to the rod at z = infinity? N/C

(c) In terms of R, at what positive value of z is that magnitude maximum? R

(d) If R = 4.00 cm and Q = 9.00 �C, what is the maximum magnitude?N/C

Answer 1

a) E_{z} = 0, b) E_{z} = 0, c) z = 1.73 [1 +
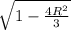`\sqrt{1 - (4R^2)/(3) }`], d)
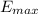`E_(max)`Emax = 9.7 10¹⁰ N / C

Step-by-step explanation:

For this exercise we use the expression

E = k ∫ dq / r²

By applying this expression to our problem of a ring of radius R, with a perpendicular axis in the z direction, we can calculate the electric field for a small charge element

dE = k dq / r²

In the attachment we can see a diagram of the electric field, it is observed that the fields perpendicular to the z axis cancel and the field remains in the direction of the axis

d
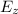`E_(z)`= dE cos φ

we substitute

E_{z} = k∫
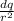`(dq)/(r^2)` cos φ

let's write the expressions

r² = R² + z²

cos φ = z / r

we substitute in the integral, where we see that the load differential does not depend on the distance and the value of the total load is + Q

E_{z} = k
`(1)/( (R^2 +z^2) ) \ (z)/( (R^2 + z^2)^(1/2) )` ∫ dq

E_{z} = k Q
`(z)/( (R2+z^2)^(3/2) )`

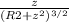
This is the expression for the electric field in the axis perpendicular to the ring, we analyze this expression to answer the questions

a) the magnitude of the field at z = 0

E_{z} = 0

b) the magnitude of the field for z = inf

when z »R the expression remains

E_{z} = k
`(z)/(z^(3) )` Q

E_{z} = k Q
`(1)/(z^2)`

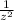
therefore when the value of z = int the field goes to E_{z} = 0

c) In value of z for which the field is maximum.

We have an extreme point when the first derivative is equal to zero

`(dE_z)/(dz ) = k Q [ (R^2 +z^2)^(3/2) - z \ 3 (z)/( (R^2 +z^2)^(1/2) ) = 0`

we solve

(R² + z²)^{ 3/2} = 3 z² /(R² +z²) ^{1/2}

(R² + z²)² = 3z²

r² + z² = √3 z

z² –1.732 z + R² = 0

we solve the quadratic equation

z = [1.732 ±
`√(3 - 4R^2)`]/ 2 = [1.73 ± 1.73
`\sqrt{ 1 - (4 R^2)/(3) }` ] / 2

z = 0.865 [1 ±
`\sqrt{1 - (4R^2)/(3) }`]

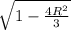Therefore there are two points where the field has an extreme point one, one is a maximum and the other a minimum, as we have already determined a minimum at z = 0 the maximum point must be

z = 0.865 [1 +
`\sqrt{1 - (4R^2)/(3) }`]

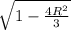d) the value of Emax

z₁ = 0.865 [1+
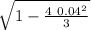`\sqrt{1 - (4 \ 0.04^2)/(3) }`) = 1.73 [1 + √0.99786]

z₁ = 1.729 m

z₂ = 0.865 [1 - √0.99786 ]

z₂ = 0.0011 m≈ 0

for which the field has a maximum value substituting in equation 1

`E_(max) = 9 10^9 \ 9 \ (1.729)/((0.04^2 + 1.729^(3/2)))`

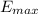`E_(max)` = 81 10⁹
`(1.729)/(1.4408)`

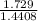
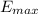`E_(max)`Emax = 9.7 10¹⁰ N / C