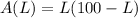Question

A rectangle has perimeter 200 feet.

1. Let L represent the length of the rectangle. Write an equation for the width W in terms of L.
2. Write a function A(L) that represents the area of the rectangle with length L.​

Answer 1

Answer:1. (200-2L)/2 2.L x (200-2L)/2=A

Explanation:

Answer 2

Part 1)

`W=100-L`

Part 2)

`A(L)=L(100-L)`

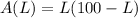
Step-by-step
`W = 100-L` explanation:

We are given a rectangle with a perimeter of 200 feet.

Part 1)

The formula for perimeter for a rectangle is:

`200=2(L+W)`

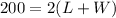
We can divide both sides by 2:

`100=L+W`

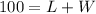
And subtracting L from both sides yields:

`W=100-L`

Part 2)

The area of a rectangle is given by:

`A(L)=LW`

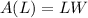
Since we solved for W earlier:

`A(L)=L(100-L)`