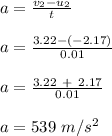Answer:
(a) the unknown inertia is 0.388 kg
(b) the average acceleration of the heavier cart is 317 m/s²
(c) the average acceleration of the lighter cart is 539 m/s²
Step-by-step explanation:
Given;
mass of the first cart, m₁ = 0.66 kg
initial speed of the first cart, u₁ = 1.85 m/s
let the mass of the cart with unknown inertia be m₂
initial velocity of the second cart, u₂ = 2.17 m/s to the left
velocity of the first cart after collision, v₁ = 1.32 m/s to the left
velocity of the second cart after collision, v₂ = 3.22 m/s
time of collision, t = 0.010 s
(a) What is the unknown inertia?
Apply the principle of conservation of linear momentum, to determine the unknown inertia.
let leftward direction be negative direction
let rightward direction be positive direction
m₁u₁ + m₂u₂ = m₁v₁ + m₂v₂
0.66(1.85) + m₂(-2.17) = 0.66(-1.32) + m₂(3.22)
1.221 - 2.17m₂ = -0.8712 + 3.22m₂
1.221 + 0.8712 = 3.22m₂ + 2.17m₂
2.0922 = 5.39m₂
m₂ = 2.0922 / 5.39
m₂ = 0.388 kg
The unknown inertia is 0.388 kg
(b) the average acceleration of the heavier cart
the heavier cart has a mass of 0.66 kg

(c) the average acceleration of the lighter cart;
the lighter cart has a mass of 0.388 kg