Question

Choose the graphic representation of the quadratic function f(x)=x^2-8x+24

Answer 1

The Answer is ----D----

Answer 2

Refer the attached figure.

Explanation:

Given : The quadratic equation -
`f(x)=x^2-8x+24`

To find : The graphic representation of the quadratic function ?

Solution :

We plot the graph of quadratic equation,

The standard form of equation is
`y=ax^2+bx+c`

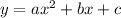
Comparing with given equation,
`f(x)=x^2-8x+24`

a=1 , b=-8 , c=24

Axis of symmetry is
`x=-(b)/(2a)`

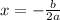
The axis of symmetry of given equation is
`x=-(-8)/(2(1))=4`

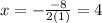
The vertex form of quadratic equation is
`f (x) = a(x - h)^2 + k`

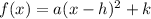
Where, (h,k) are the vertex.

Convert the quadratic equation into vertex form,

By completing the square,

`f(x)=x^2-8x+24`

`f(x)=(x^2-2(4)x+(4)^2)-(4)^2+24`

`f(x)=(x-4)^2+8`

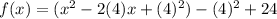
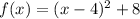
On comparison,

(h,k)=(4,8)

Now, we plot the equation with vertex (4,8).

Refer the attached figure below.