Question

select all of the correct answers for the values of x in each of the angles D, E and C in the following regular heptagon:

Answer 1

Since we have here a regular heptagon, we have that the sum of the inner angles of this regular polygon is given by the formula:

(n - 2) * 180

We have that n = 7, then the sum of the inner angles is (7 - 2) * 180 = 5 * 180 = 900.

Since in this regular polygon, the angles and the sides are the same, then we have that the measure of each angle is:

900/7 = 128.571 degrees.

Then, we need to take each of the given equations and equate them to the latter value. Therefore, we have:

First Case:

5x + 5 = 128.571 ---> x = 24.7142

We have this value, because:

`5x+5=128.571\Rightarrow5x=(128.571-5)\Rightarrow x=((123.571))/(5)\Rightarrow x=24.7142`

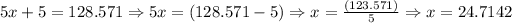
Second Case:

7x + 1 = 128.571 ---> x = 18.2244

We have this value, because:

`7x+1=128.571\Rightarrow7x=128.571-1\Rightarrow x=(127.571)/(7)\Rightarrow x=18.2244`

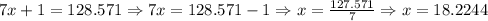
Third Case:

x = 36.1631.

And this value is the result of:

`(7)/(2)x+2=128.571\Rightarrow(7)/(2)x=128.57-2=126.571\Rightarrow x=(2)/(7)\cdot126.571=36.1631`

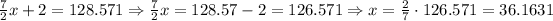
Therefore, we have that:

x = 24.7142 in

x = 18.2244 in

x = 36.1631 in < C (option 2)