Question

Find the area of triangle ABC.A. 13.1 units²B. 21.61 units²C. 30.3 units²D. 14.65 units²

Answer 1

The area of triangle ABC given the angles and sides is 14.65 units ². option D

What is the area of the triangle?

Angle A = 84.08°

Angle B = 62.86°

b = 6.93

c = 4.25

So,

Area of the triangle = ½ × b × c × sin A

= ½ × 6.93 × 4.25 × sin 84.08°

= ½ × 29.4525 × 0.994666875152874

= 14.64771307022001

Approximately,

14.65 square units

Therefore, the approximate area of the triangle is 14.65 units².

Answer 2

Given in the following figure:

AB = 4.25

AC = 6.93

∠A = 84.08°

∠B = 62.86°

With the following given, for us to be able to get the area of the triangle, we will be using the following formula:

`\text{ Area = }\frac{\text{ ab x sin(}\angle\text{C)}}{\text{ 2}}`

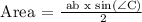
Where,

a and b are two adjacent sides of the triangle and ∠C is the angle between the two sides.

From the given, AB and AC are adjacent sides and the angle between them is ∠A.

Now, from the formula that we will be using, let:

a = AB = 4.25

b = AC = 6.93

∠C = ∠A = 84.08°

We get,

`\text{ Area = }\frac{\text{ ab x sin(}\angle\text{C)}}{\text{ 2}}`
`\text{ = }\frac{(4.25)(6.93)\text{ x sin (}84.08\degree)}{2}`
`\text{ = }\frac{(29.4525)\text{ x sin (}84.08\degree)}{2}`
`\text{ = (}14.72625)\text{ x sin (}84.08\degree)`
`\text{ Area = 14.647713 }\approx\text{ 14.65 sq. units}`

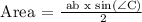
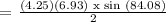
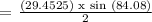
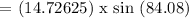
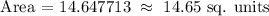
Therefore, the answer is letter D.