Question

What transformation has changed the parent function f(x) = 3(2)x to its new appearance shown in the graph below?

exponential graph passing through point 2, 3.

Answer 1

if you mean -2 and 3. than the answer would be f(x +2)

Answer 2

The transformed function is:

g(x)=f(x-2)

Explanation:

We are given a parent function f(x) as:

`f(x)=3* 2^x`

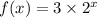
Now some transformation is appplied to the function f(x) such that the transformed function is a exponential function that passes through the point (2,3).

Let the transformed function be denoted by g(x).

Hence, the transformation is:

g(x)=f(x-2)

This implies

`g(x)=3* 2^(x-2)`

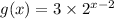
Since, at x=2 we have:

`g(x)=3* 2^(2-2)\\\\g(x)=3* 2^0\\\\g(x)=3`

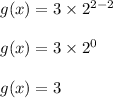
Hence, the function passes through the point (2,3)