Question

Consider f(x)= -(x+7)^2+4

Answer 1

1.) Yes, we know it is a quadratic as it is being squared

2.) The graph isn't linear because it's quadratic

3.) f(x)= -(x+7)^2+4 is already in vertex form, a(x - h)^2 + k (emphasis on the a(x-h) because you the vertex is not -h, but just h) where (h, k) is the vertex. That means the vertex should be (-7, 4), not (7, 4)

4.) The formula for the axis of symmetry is x = -b/(2a). Convert this from vertex to standard form (ax^2 + bx + c) and you should get x = -7, so yes, that is correct.

5.) To find the y-intercept, plug in zero for x. You should get -45. That means the y-intercept is (0, -45), not (0, 4).

6.) Yes, the graph does have a higher section. It's the vertex.

7.) To determine the number of real solutions, you convert this to standard form, and plug in the values of a, b, and c into the quadratic formula (-b +/- √b^2 - 4ac/(2a)) Look at the discriminant or the number under the radical, b^2 - 4ac. If it is a positive number, there are two real solutions, if it is negative, there will be no real solutions, but rather two complex solutions. If it is equal to 0, there is one real solution.
In this case, there are two real solutions, not no real solutions.

Answer 2

1,4,6 are true statements.

Explanation:

Given :
`f(x)= -(x+7)^2+4`

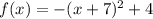
To find : Which of the following are true

Solution :

1) The equation is quadratic.

Yes it is true as the given equation is quadratic as (x+7) is squared.

2) The graph is linear

No it is not true as the graph is not linear because it is quadratic.

3) The vertex is (7,4)

The general form of vertex is
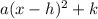`a(x - h)^2 + k` where h,k is the vertex.

In the given equation (-7,4) is the vertex of the given equation.

So, No it is not true

4)The axis of symmetry is x=-7

The formula for the axis of symmetry is
`x=-(b)/(2a)`

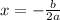
Convert it in general quadratic form we get
`-x^2-14x-45`

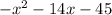
where a=-1,b=-14,c=-45

Vertex is
`x=-(-14)/(2(-1))=-7`

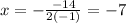
Yes it is true.

5) The y-intercept is (0,4).

To find y-intercept put x=0

`f(x)= -(x+7)^2+4`

`f(x)= -(0+7)^2+4=-49+4=-45`

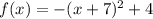
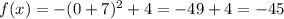
So, y-intercept is (0,-45)

No it is not true.

6) The graph has relative maximum.

Yes it is true, the graph has a higher section i.e, vertex.

7) The equation has no real solution.

To find the solution we have to find the discriminant

`D=b^2-4ac`

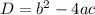
Quadratic form of given equation is
`-x^2-14x-45`

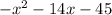
where a=-1,b=-14,c=-45

`D=(-14)^2-4(-1)(45)`

`D=196+180`

`D=376`

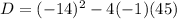
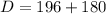
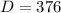
If D is a positive number, there are two real solutions.

Yes it is true.