Question

A spinner is divided into eight equal parts numbered 1 to 8. If the spinner spun once, what is the probability that it stops on a number that is a multiple of 2?

Answer 1

probability=total of even number/total of all numbers

P(e)=4/8=1/2

Answer 2

`\bf\implies \textbf{Hence, The required probability = }(1)/(2)`

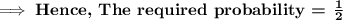
Explanation:

A spinner is divided into eight equal parts numbered 1 to 8.

Now, The spinner is spun once.

Total number of outcomes on spinning the spinner once = 8

We need to find the probability that it stops on a number that is a multiple of 2.

So, Multiples of 2 from 1 to 8 : 2, 4, 6 and 8

Total number of favorable outcomes = 4

`\text{Hence, The required probability = }(4)/(8)\\\\\bf \implies \textbf{Hence, The required probability = }(1)/(2)`