Ok, so
We want to find an odd degree polynomial but is NOT an odd function.
This polynomial could be:
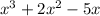
This is an odd degree polynomial because the highest degree is 3 which is an odd number.
Now, we're going to check that this isn't an odd function.
An odd function is supposed to satisfy that:
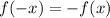
So, we're going to check this with our example:
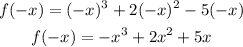
Now, let's make - f(x):
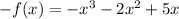
As you can see, these expressions aren't the same.
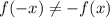
Therefore, the function is not odd.