Question

Graph Each pair of inequalities and indicate the solution set of the system with crosshatching or shading

Answer 1

Step-by-step explanation:

Given:

`\begin{gathered} x-3y\leq12 \\ x>2 \end{gathered}`

To find:

The graph of the inequalities and indicating the solution set of the system with crosshatching or shading

Recall that the slope-intercept form of the equation of a line is generally given as;

`\begin{gathered} y=mx+b \\ where\text{ }m=slope\text{ of the line} \\ \text{ b }=y-intercept\text{ of the line} \end{gathered}`

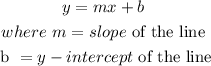
Let's rewritethe first inequality yin slope-intercept form using as seen below;

`\begin{gathered} x-3y\leq12 \\ -3y\leq-x+12 \\ (-3y)/(-3)\leq(-x)/(-3)-(12)/(3) \\ y\ge(1)/(3)x-4 \end{gathered}`

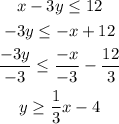
We can see from theabove that the slope (m)of the line is 1/3 and the y-intercept isi -4.

Since the inequality has a greater than sign, we'll shade the region above the line.

Also since the inqua;lity has an equal sign, the line will be solid.

See below the graph of the inequality;

For the second inequality, the line will be dashed since it does not have an equal sign. We'll shade the region to the right of the line since it has a greater than sign.

See below the graph of the inequality;

We will now go ahead and combine the two graphs, where thetwo shaded regions intersec isrepresent the solution set of the system as seen below;