Question

The Period of a function is 4pi.

How many cycles of the function occur in a horizontal length of 12pi?

Answer 1

3 pi

Explanation:

12pi / 4pi = 3pi

hope it helps :)

Answer 2

3 cycles of the function occur in a horizontal length of 12π with period of function 4π

Explanation:

Given the period of function 4π.

we have to find the number of cycles of function occur in a horizontal length of 12π.

As, the total horizontal length of function is equals to number of cycles into the period of function.

L=(no. of cycles)P

where L is length of horizontal line and P is the period of function.

Hence,

`\text{No. of cycles=}(L)/(P)=(12\pi)/(4\pi)=3`

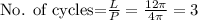
∴ 3 cycles of the function occur in a horizontal length of 12π with period of function 4π