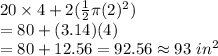Question

The figure below shows two half-circles at the ends of a rectangle with the dimensions shown. Which is closest to the area of the figure in square inches.

a 93
b 130
c 86
d 105

Answer 1

Answer: a 93

Explanation:

From the given figure, it can be seen that there are one rectangle having dimensions
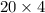`20*4` and two semicircle with same diameter of 4 inches.

Radius of semicircles =
`(4)/(2)=2\ in.`

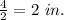
Area of semicircle =
`(1)/(2)\pi r^2`

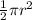
Now, the area of the figure=Area of rectangle+Area of two semicircles

=
`20*4+2((1)/(2)\pi (2)^2)\\=80+(3.14)(4)\\=80+12.56=92.56\approx93\ in^2`