Question

A farmer is planning a rectangular area for her chickens. The area of the rectangle will be 800 square feet. Three sides of the rectangle will be formed by fencing, which costs $5 per foot. The fourth side of the rectangle will be formed by a portion of the barn wall, which requires no fencing. In order to minimize the cost of the fencing, how long should the fourth side be?

Answer 1

40 feet.

Explanation:

Area of rectangle = 800 square feet

Let the fourth side of the rectangle be x.

Length of the rectangle = x feet

The area of a rectangle is

`A=length* width`

`800=x* width`

`(800)/(x)=width`

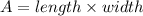
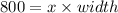
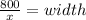
Cost of fencing = $5 per foot

Cost function on three sides is

`C(x)=5[length+2(width)]`

`C(x)=5x+10((800)/(x))`

`C(x)=5x+(8000)/(x)`

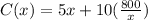
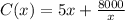
Differentiate with respect to x.

`C'(x)=5-(8000)/(x^2)`
`[\because (d)/(dx)((1)/(x))=-(1)/(x^2)]`

To find the critical point equate C'(x)=0.

`0=5-(8000)/(x^2)`

`-5=-(8000)/(x^2)`

`-5x^2=-8000`

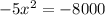
Divide both sides by -5.

`x^2=-(8000)/(-5)`

`x^2=1600`

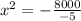
Taking square root on both sides,

`x=40`

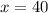
Differentiate C'(x) with respect to x.

`C''(x)=(16000)/(x^3)>0`

Since C''(x)>0 for x=40, therefore cost of the fencing is minimum at x=40.

Thus, the measure of fourth side of the rectangle is 40 feet.

Answer 2

40 ft

Explanation:

Let x represent the length in feet of the fourth side. Then the sides perpendicular to the barn wall will have length 800/x, and the total cost of the fence will be ...

cost = $5 × (x + 2·800/x)

The derivative of cost with respect to x will be zero when the cost is a minimum:

d(cost)/dx = 5 -8000/x^2 = 0

5x^2 = 8000 . . . . . multiply by x^2, add 8000

x = √1600 = 40 . . . . feet . . . . . . divide by 5, take the square root

The length of the fourth side should be 40 feet.