Question

What is the answer to all parts of this question, all answers must be in 3 significant digits.

Answer 1

Answers:

a. 1.80 s

b. 0.363 m/s (10.4 cm)

0.590 m/s (16.9 cm)

c. 1.27 m/s² (10.4 cm)

2.06 m/s² (16.9 cm)

d. 0.00997 N (10.4 cm)

0.0162 N (16.9 cm)

Step-by-step explanation:

Part a.

First, let's convert 33 1/3 rpm to rad/s, so

`33.333\text{ rev/min}*\frac{2\pi\text{ rad}}{1\text{ rev}}*\frac{1\text{ min}}{60\text{ s}}=3.49\text{ rad/s}`

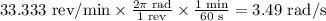
So, the angular velocity w of the record is 3.49 rad/s. Now, we can calculate the period T as follows

`T=(2\pi)/(w)=\frac{2\pi}{3.49\text{ rad/s}}=1.80\text{ s}`

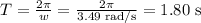
Part b.

The speed of the coin can be calculated as

v = wr

Where r is the radius, so the speed at r = 10.4 cm and at r = 16.9 cm are equal to

`\begin{gathered} v=(3.49\text{ rad/s)(0.104 m) = }0.363\text{ m/s } \\ v=(3.49\text{ rad/s)(0.169 m) = 0.590 m/s} \end{gathered}`

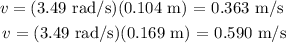
Part c.

Then, the acceleration is equal to

`a=(v^2)/(r)`

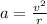
So, for each case, we get

`\begin{gathered} a=\frac{(0.363m/s)^2}{0.104\text{ m}}=1.27m/s^2 \\ a=\frac{(0.590m/s)^2}{0.169\text{ m}}=2.06m/s^2 \end{gathered}`

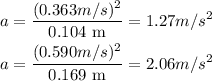
Part d.

Finally, the net force is equal to the mass times the acceleration, so for each case, we get

`\begin{gathered} F_{\text{net}}=ma \\ F_{\text{net}}=(0.00787\text{ kg)}(1.27m/s^2)=0.00997\text{ N} \\ F_{\text{net}}=(0.00787\text{ kg)}(2.06m/s^2)=0.0162\text{ N} \end{gathered}`

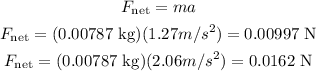
Therefore, the answers are:

a. 1.80 s

b. 0.363 m/s (10.4 cm)

0.590 m/s (16.9 cm)

c. 1.27 m/s² (10.4 cm)

2.06 m/s² (16.9 cm)

d. 0.00997 N (10.4 cm)

0.0162 N (16.9 cm)