Question

Terrence buys a new car for $20,000. The value of the car depreciates by 15% each year. If f(x) represents the value of the car after x years, which function represents the car’s value?

f(x) = 20,000(0.85)x

f(x) = 20,000(0.15)x

f(x) = 20,000(1.15)x

f(x) = 20,000(1.85)x

Answer 1

F(x)=20000(1-0.15)^x

f(x) = 20,000(0.85)^x.....answer a

Answer 2

The answer is A.
`f(x)=20,000*(0.85)^x`

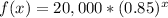
Explanation:

The base price of the car is $20,000. Then, every year, the value of the car depreciates by 15%, that it means, every year the value of the car is 85% than the value of the previous year.

If we repeat the process, for example in 4 years, the value of the car will be:

20,000*0.85*0.85*0.85*0.85

You have to consider the value of the previous year to applicate the 85%.

So, the correct function to represent the car's value is:

`f(x)=20,000*(0.85)^x`

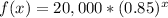
I have attached an image that shows a graph of the correct function.