Question

An open–top box is being designed by cutting a corner piece out of a 16" by 14" piece of metal and folding the sides upwards. The designer wants to maximize the volume of this box.

Answer 1

I hope you're in calculus doing this problem(?) If you cut away from both the length and the width, your sides have values then of 16 - 2x, and 14 - 2x, and the height is x. Solving this gives you a cubic: 4x^3 - 60x^2 + 224x. If you take the derivative of this function, you will get where the max value of the function occurs, since that what the derivative tells you...either a max or a min value of a function. The derivative is 12x^2 - 120x + 224. If you use the quadratic formula and solve for x, you get 2 values: 7.5 and 2.48. Sub both those values back in to the original function to get that an x value of 7.5 plugged into the original gives you a -7.5. Well, we both know that volume cannot be a negative value, so sub in the x value of 2.48 to get the max volume of 247.507968 inches cubed.

Answer 2

The strip of 16 by 14 inches.

Let x be the corner of the square cut

Then the box would have height as x, length 16-2x and width 14-2x

Hence volume =
`x(16-2x)(14-2x)\\= 4x^3-60x^2+224x`

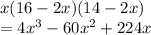
Use derivative to test to find x for maximum volume

V'(x) =
`12x^2-120x+224`

v"(x) =
`24x-120`

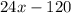
Equate first derivative to 0

Solutions are

x= 2.483 and x = 7.517

Practically cutting more than 7 inches is not possible from 14 inches dimention

Hence 2.483 is the side of square and maximum volume

= 247.508