Answer:
The median number of shoes for the girls is greater than the median number of shoes for the boys.
Explanation:
Jeffrey surveys the 13 girls and 13 boys in his class to find out how many pairs of shoes they have in their closets
Girls Data :10, 12, 9 , 15, 25,8, 6,14, 18 , 11 , 9 , 13 , 15
Arrange the data in the ascending order :
6,8,9,9,10,11,12,13,14,15,15,18,25
No. of observations n = 13(odd)
So, Median =
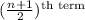
=
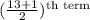
=
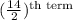
=
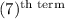
=
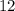
Thus the median of the girls data is 12.
Now to find
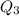
Consider the set of values right to the median .
13,14,15,15,18,25
Now find the median of this data
No. of observations n = 6(even)
Median =
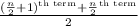
So, Median =
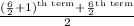
Median =
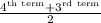
Median =

Median =
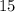
Mean =
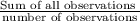
Mean =

Mean =
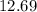
So,
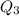 for girls is 15 and median is 12 and mean is 12.6.
for girls is 15 and median is 12 and mean is 12.6.
Boys data : 8,6,4,5,10,15,7,8,12,11,9,5,4
Arrange the data in the ascending order :
4,4,5,5,6,7,8,8,9,10,11,12,15
No. of observations n = 13(odd)
So, Median =
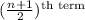
=
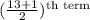
=
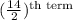
=
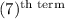
=
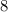
Thus the median of the boys data is 8
Now to find
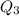
Consider the set of values right to the median .
8,9,10,11,12,15
Now find the median of this data
No. of observations n = 6(even)
Median =
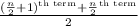
So, Median =
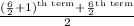
Median =
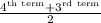
Median =
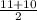
Median =
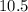
Mean =
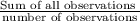
Mean =
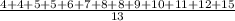
Mean =
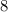
So,
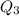 for boys is 10.3 and median is 8 and mean is 8
for boys is 10.3 and median is 8 and mean is 8
Since we can see that the value of
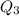 , mean and median opf girls is greater than boys.
, mean and median opf girls is greater than boys.
So, Option C is correct.
The median number of shoes for the girls is greater than the median number of shoes for the boys.