ANSWER: 32 five-dollar bills
======
Step-by-step explanation:
Let x be number of $5 bills
Let y be number of $10 bills
Since we have total of 38 bills, we must have the sum of x and y be 38
x + y = 38 (I)
Since the total amount deposited is $220, we must have the sum of 5x and 10y be 220 (x and y are just the "number of" their respective bills, so we multiply them by their value to get the total value):
5x + 10y = 220 (II)
System of equations:

Divide both sides of equation (II) by 5 so our numbers become smaller
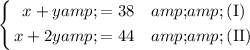
Rearrange (I) to solve for y so that we can substitute into (II)
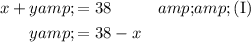
Substituting this into equation (II) for the y:
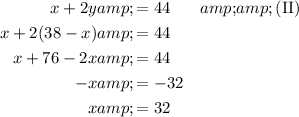
We have 32 five-dollar bills
======
If we want to finish off the question, use y = 38 - x to figure out number of $10 bills
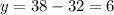
32 five-dollar bills and 6 ten-dollar bills