Question

The lengths of these sides of a triangle are 14cm, 22cm and 30cm. Find the measure of the smallest angle in the triangle to the nearest tenth.

Answer 1

As per given by the question,

There are given that a length of three sides of the triangle.

The length of sides are, 14cm, 22cm, and 30cm.

Now,

For finding the measure of the smallest angle,

The smallest angle in a triangle is always opposite the shortest side. and also the bigest angle is always opposite the longest side.

So,

Suppose length A is 14cm, B is 22 cm and C is 30cm.

Then,

From the law of cosines,

Let A be the smallest angle.

So,

`14^2=30^2+22^2-2(30)(22)\cos A`

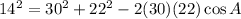
Now,

Find the value of angle A,

`\begin{gathered} 14^2=30^2+22^2-2(30)(22)\cos A \\ 196=900+484-1320\cos A \end{gathered}`

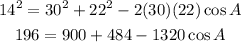
Now,

`\begin{gathered} 196=1384-1320\text{ cosA} \\ 196-1384+1320\cos A=0 \\ -1188+1320\cos A=0 \\ -1188=-1320\cos A \\ \cos A=(1188)/(1320) \end{gathered}`

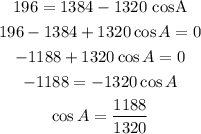
Then,

`\begin{gathered} \cos A=(1188)/(1320) \\ \cos A=0.9 \\ A=\cos ^(-1)(0.9) \\ A=25.84^(\circ) \end{gathered}`

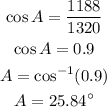
Hence, the measure of the smallest angle is 25.84 degree.