Question

A ball is thrown vertically upward. After t seconds, it’s height h(in feet) is given by the function h(t)=44t-16t^2. What is the maximum height that the ball will reach? Do not round

Answer 1

Given

`h\mleft(t\mright)=44t-16t^(2.)`

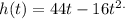
Find

the maximum height that the ball will reach

Step-by-step explanation

The maximum of the function can be found by equating its first derivative to zero.

so, we need to find the first derivative of the function.

`\begin{gathered} h\mleft(t\mright)=44t-16t^(2.) \\ h^(\prime)(t)=44-32t \end{gathered}`

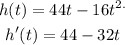
put h'(t) = 0

`\begin{gathered} 44-32t=0 \\ 32t=44 \\ t=(44)/(32) \\ \\ t=(11)/(8) \end{gathered}`

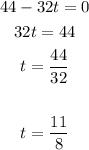
so , the maximum height is found by substituting the value of t into the original equation

`\begin{gathered} h(t)=44((11)/(8))-16((11)/(8))^2 \\ \\ h(t)=60.5+30.25 \\ h(t)=30.25 \end{gathered}`

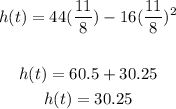
Final Answer

the maximum height that the ball will reach is 30.25 feet