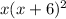Question

Which of the following is the correct factorization of the polynomial below?

x3 + 12x2 + 36x

Answer 1

D is the answer. x(x^2+12x+36)
= x(x+6)^2

Answer 2

Option (d) is correct.

The correct factorization of the polynomial
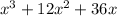`x^3+12x^2+36x` is
`x(x+6)^2`

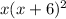
Explanation:

Given : Polynomial
`x^3+12x^2+36x`

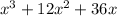
We have to factorize the given polynomial
`x^3+12x^2+36x`.

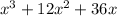Consider the given equation
`x^3+12x^2+36x`

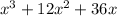
Taking x common from each term, we have,

`x^3+12x^2+36x=x(x^2+12x+36)`

Rewrite 36 as
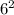`6^2` , we get,

`x(x^2+12x+36)=x(x^2+12x+6^2)`

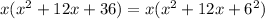
Using Algebraic identity,
`\left(a+b\right)^2=a^2+2ab+b^2`

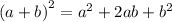
We have,

`a=x,\:b=6`

`x(x^2+12x+6^2)=x(x+6)^2`

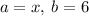
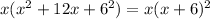
Thus, The correct factorization of the polynomial
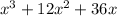`x^3+12x^2+36x` is
`x(x+6)^2`