we know that
The equation of a vertical parabola in vertex form is equal to
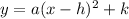
where
(h,k) is the vertex of the parabola
if
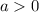 -----> the parabola open upward (vertex is a minimum)
-----> the parabola open upward (vertex is a minimum)
if
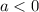 -----> the parabola open downward (vertex is a maximum)
-----> the parabola open downward (vertex is a maximum)
In this problem we have
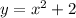
The vertex is the point
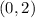
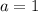
so
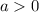 -----> the parabola open upward (vertex is a minimum)
-----> the parabola open upward (vertex is a minimum)
Using a graphing tool
see the attached figure
The answer is
The vertex is the point
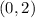
Is a minimum