Question

What are the possible values of a and b?

Answer 1

`\bf a^{\frac{{ n}}{{ m}}} \implies \sqrt[{ m}]{a^( n)} \qquad \qquad \sqrt[{ m}]{a^( n)}\implies a^{\frac{{ n}}{{ m}}}\\\\ -----------------------------\\\\ 7^{(19)/(4)}\cdot \sqrt[a]{7^b}=7^{(9)/(4)}\cdot √(7^3)\implies 7^{(19)/(4)}\cdot \sqrt[a]{7^b}=7^{(9)/(4)}\cdot \sqrt[2]{7^3} \\\\\\ 7^{\cfrac{}{}(19)/(4)}\cdot 7^{\cfrac{}{}(b)/(a)}=7^{\cfrac{}{}(9)/(4)}\cdot 7^{\cfrac{}{}(3)/(2)}\implies 7^{\cfrac{}{}(19)/(4)+(b)/(a)}=7^{\cfrac{}{}(9)/(4)+(3)/(2)}`


`\bf \textit{same base, thus, the exponents must be the same} \\\\\\ \cfrac{19}{4}+\cfrac{b}{a}=\cfrac{9}{4}+\cfrac{3}{2}\implies \cfrac{b}{a}=\cfrac{9}{4}+\cfrac{3}{2}-\cfrac{19}{4}\implies \cfrac{b}{a}=\cfrac{9+6-19}{4} \\\\\\ \cfrac{b}{a}=\cfrac{-4}{4}\implies \cfrac{b}{a}=\cfrac{-1}{1}\implies \begin{cases} b=-1\\ a=1 \end{cases}`

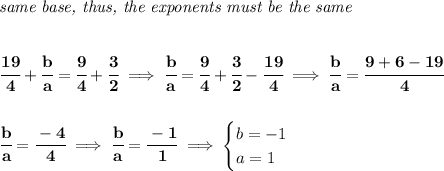
now... if you multiply the numerator and denominator by some same number, the fraction still holds true, for example -3/3 simplified is just -1/1
or -1,000,000/1,000,000 simplified is also -1/1

so.. .the possible values, can be anything, so long the numerator and denominator maintain that ratio