Question

Use the Comparison Theorem to determine whether the integral is convergent or divergent.

Answer 1

First, examine what happens over the integration domain.


`\displaystyle(\sin^2x)/(\sqrt x)=x^(3/2)(\sin^2x)/(x^2)\implies\lim_(x\to0^+)x^(3/2)(\sin^2x)/(x^2)=0*1=0`


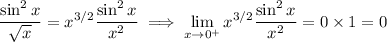
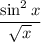
`(\sin^2x)/(\sqrt x)` is continuous for
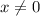
`x\\eq0`, so we don't need to worry about the right endpoint.

Now, note that


`(\sin^2x)/(\sqrt x)\le\frac1{\sqrt x}`

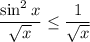
due to the fact that
`|\sin x|\le1\implies0\le\sin^2x\le1`. So, if the larger integral converges, so must the smaller one. You have


`\displaystyle\int_0^\pi(\mathrm dx)/(\sqrt x)=\lim_(t\to0^+)\int_t^\pi x^(-1/2)\,\mathrm dx`

`=\displaystyle\lim_(t\to0^+)2x^(1/2)\bigg|_(x=t)^(x=\pi)=2\left(\sqrt\pi-\lim_(t\to0^+)\sqrt t\right)=2\sqrt\pi`

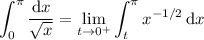
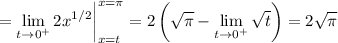
so the larger integral converges. Therefore the smaller one does, too.