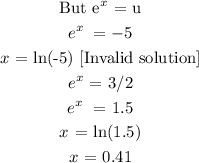Question

The given equation is quadratic in form. Solve the following equation and give exact solutions.2e^(2x)+7e^(x)=151. The equation rewritten as a quadratic equation in standard form using u-substitution is?2. What is the exact solution?Write an exact answer in simplified form.

Answer 1

Answer:

1) The equation rewritten as a quadratic equation in standard form using u-substitution is:

2u² + 7u - 15 = 0

2) The exact solution is:

x = 0.41

Explanations:

The qiven equation is:

`2e^(2x)+7e^x\text{ = 15}`

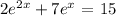
This can be rewritten as:

`2(e^x)^2+7e^x\text{ - 15 = 0}`
`\text{Let u = e}^x`

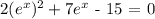
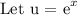
The equation rewritten as a quadratic equation in standard form becomes:

`2u^2\text{ + 7u - 15 = 0}`

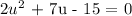
Factorize the quadratic equation above:

`\begin{gathered} 2u^2\text{ - 3u + 10u - 15 = 0} \\ u\text{ (2u - 3) + 5(2u - 3) = 0} \\ (u\text{ + 5) (2u - 3) = 0} \\ u\text{ + 5 = 0} \\ u\text{ = -5} \\ 2u\text{ - 3 = 0} \\ 2u\text{ = 3} \\ u\text{ = }(3)/(2) \end{gathered}`

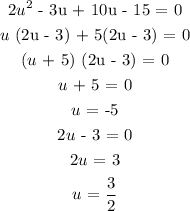
u = -5 or u = 3/2

`\begin{gathered} \text{But e}^x\text{ = u} \\ e^{x\text{ }}=-5^{} \\ x\text{ = ln(-5) \lbrack{}Invalid solution\rbrack} \\ e^x\text{ = 3/2} \\ e^{x\text{ }}\text{ = 1.5} \\ x\text{ = ln(1.5)} \\ x\text{ = }0.41 \end{gathered}`