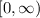Question

What is the domain and range for the following function and its inverse?

f(x) = x2 + 3

Answer 1

I assume you mean  x^2  + 3. For the function x^2 + 3, the domain is (-infinity,infinity) and the range is (3,infinity). The function's inverse is +- sqrt(-3 + x).

Answer 2

Domain of f:R

Range of f:
`[3,\infty)`

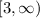
Domain of inverse of f:
`[3,\infty)`

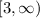
Range of inverse of f:
`[0,\infty)`

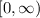
Explanation:

We are given that a function

`f(x)=x^2+3`

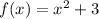
Function is defined for every value of x

Therefore, domain of function is R.

Substitute x=0 then we get

`f(0)=3`

Therefore, range of given function is (3,
`\infty`).

Suppose, f(x)=y=
`x^2+3`

`x=√(y-3)`

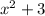
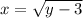
Function is not defined for negative values of x and for 1 and 2.

Therefore, the domain of inverse function of given function is
`[3,\infty)`.

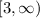When substitute y=3 then we get

x=0

Therefore, the range of given function is
`[0,\infty)`