Answer:
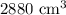 .
.
Explanation:
We have been that a container is shaped like a cylinder with half spheres on each end. The cylinder has a length of 30 centimeters and a radius is 5 centimeters.
To find the volume of container we will use volume of container and volume of sphere formula.
Since half spheres are on the each side of cylinder, so adding them we will get one sphere.
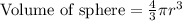 , where r represents radius of sphere.
, where r represents radius of sphere.

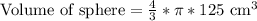
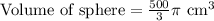
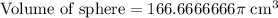
 , where r represents radius and h represents height of cylinder.
, where r represents radius and h represents height of cylinder.
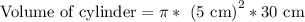
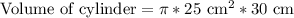
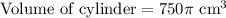

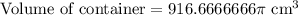
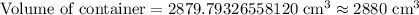
Therefore, the volume of our given container is
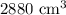 .
.