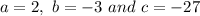Question

Donnie is solving the equation 2x^2=27+3x with the quadratic formula. What values should he use for a , b , and c? A. a=2 b=27 c=3 B. a=2 b=-27 c=-3 C. a=2 b=3 c=27 D. a=2 b=-3 c=-27

Answer 1

The value of a,b and c is:

`a=2,\ b=-3\ \text{and}\ c=-27`

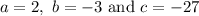
Explanation:

We know that any quadratic equation is given by the form:

`ax^2+bx+c=0`

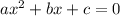
and the formula which gives the solution of the equation is given by:

`x=(-b\pm √(b^2-4ac))/(2a)`

Here we have the equation as:

`2x^2=27+3x`

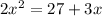
which is also given by:

`2x^2-(27+3x)=0\\\\i.e.\\\\2x^2-27-3x\\\\2x^2-3x-27=0`

i.e. on comparing with the general quadratic formula we have:

`a=2,\ b=-3\ and\ c=-27`