Answer:
Option A

Explanation:
we know that
The Triangle Inequality Theorem states that the sum of any 2 sides of a triangle must be greater than the measure of the third side
Verify each case
case A) we have

we know that
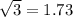
Applying the Triangle Inequality Theorem
1) 5+6 > 1.73 -----> is true
2) 5+1.73 > 6 ----> is true
therefore
The side lengths can represent the sides of a triangle
case B) we have
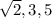
we know that

Applying the Triangle Inequality Theorem
1) 1.41+3 > 5 -----> is not true
therefore
The side lengths can not represent the sides of a triangle
case C) we have
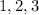
Applying the Triangle Inequality Theorem
1) 1+2 > 3 ----> is not true
therefore
The side lengths can not represent the sides of a triangle
case D) we have
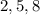
Applying the Triangle Inequality Theorem
1) 2+5 > 8 ----> is not true
therefore
The side lengths can not represent the sides of a triangle