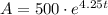Question

Elaine deposited $500 at 4.25% compounded continuously for t number of years.

Answer 1

`A=500*(1+ (4.25)/(100) )^t`

Hope this helps!!

Answer 2

`A = 500 \cdot e^(4.25t)`

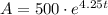
Explanation:

Using the formula for continuously compounded interest is given by:

`A = Pe^(rt)`

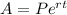
where

P is the present value

A is the future value

r is the rate and

t is the time in years respectively.

As per the statement:

Elaine deposited $500 at 4.25% compounded continuously for t number of years.

⇒
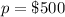`p = \$500`, r = 4.25%

Substitute these in [1] we have;

`A = 500 \cdot e^(4.25t)`

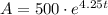
Therefore, Elaine deposited $500 at 4.25% compounded continuously for t number of years is:

`A = 500 \cdot e^(4.25t)`