Question

An RC car is carrying a tiny slingshot with a spring constant of 85 N/m at 0.2 m off the ground at 5.6 m/s. The sling shot is pulled back 3.5 cm from a relaxed state and shoots a 25 g steel pellet in the same direction the car is moving. What is the velocity of the steel pellet relative to the ground as it leaves the sling shot

Answer 1

The velocity of the steel pellet relative to the ground when it leaves the sling shot is approximately 5.960 meters per second.

Step-by-step explanation:

Let suppose that RC car-slingshot-steelpellet is a conservative system, that is, that non-conservative forces (i.e. friction, air viscosity) can be neglected. The velocity of the steel pellet can be found by means of the Principle of Energy Conservation and under the consideration that change in gravitational potential energy is negligible and that the RC car travels at constant velocity:

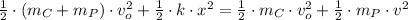
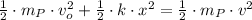
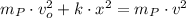
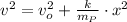
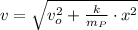`(1)/(2)\cdot (m_(C)+m_(P))\cdot v_(o)^(2) + (1)/(2)\cdot k\cdot x^(2) = (1)/(2)\cdot m_(C)\cdot v_(o)^(2) + (1)/(2)\cdot m_(P)\cdot v^(2)`

`(1)/(2)\cdot m_(P)\cdot v_(o)^(2) + (1)/(2)\cdot k\cdot x^(2) = (1)/(2)\cdot m_(P)\cdot v^(2)`

`m_(P)\cdot v_(o)^(2) + k\cdot x^(2) = m_(P)\cdot v^(2)`

`v^(2) = v_(o)^(2) + (k)/(m_(P))\cdot x^(2)`

`v = \sqrt{v_(o)^(2)+(k)/(m_(P))\cdot x^(2) }` (1)

Where:

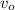`v_(o)` - Initial velocity of the steel pellet, measured in meters per second.

`v` - Final velocity of the steel pellet, measured in meters per second.

`k` - Spring constant, measured in newtons per meter.

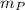`m_(P)` - Mass of the steel pellet, measured in kilograms.

`m_(C)` - Mass of the RC car, measured in kilograms.

`x` - Initial deformation of the spring, measured in meters.

If we know that
`v_(o) = 5.6\,(m)/(s)`,
`k = 85\,(N)/(m)`,
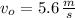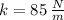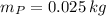`m_(P) = 0.025\,kg` and
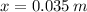`x = 0.035\,m`, then the velocity of the steel pellet relative to the ground when it leaves the sling shot is:

`v = \sqrt{\left(5.6\,(m)/(s) \right)^(2)+(\left(85\,(N)/(m) \right)\cdot (0.035\,m)^(2))/(0.025\,kg) }`

`v \approx 5.960\,(m)/(s)`

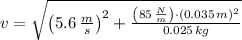
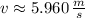
The velocity of the steel pellet relative to the ground when it leaves the sling shot is approximately 5.960 meters per second.