The number of letters in the word MATH IS
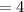
To rearrange a letter with n letters to form a four-letter code will be
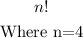
Hence,
The number of ways of rearranging the words will be
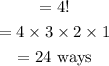
This problem is a bit different. Instead of choosing one item from each of several different
categories, we are repeatedly choosing items from the same category (the category is: the
letters of the word MATH) and each time we choose an item we do not replace it, so there is
one fewer choice at the next stage: we have 4 choices for the first letter (say we choose A),
then 3 choices for the second (M, T, and H; say we choose H), then 2 choices for the next
letter (M and T; say we choose M) and only one choice at the last stage (T). Thus, there are
4 · 3 · 2 · 1 = 24 ways to spell a code word with the letters MATH.
Hence,
The final answer = 24 ways