Let
x-------> the amount of
 solution
solution
y--------> the amount of
 solution
solution
we know that
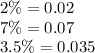
so

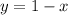 -------> equation A
-------> equation A
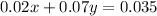 -------> equation B
-------> equation B
substitute equation A in equation B
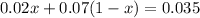
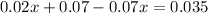
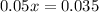
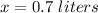
find the value of y
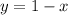

therefore
The student need
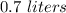 of
of
 solution and
solution and
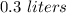 of
of
 solution
solution
the answer is
A) The percent values were written incorrectly in the equation
B) The amount of 7% solution should be written as 1 – x, not x – 1.