State the parameters given in the question
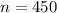
The sample size n is 450
Hence, the first box n=450
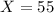
The number of students X that attended private school is 55
Hence, the second box X=55
Therefore, sample proportion is

Hence, the third box is 'p=0.122
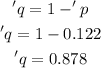
Hence, the forth box is 'q= 0.878
From the question, the confidence interval is 95% can be represented as
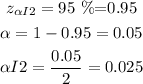
The Z score for confidence interval of 95% is gotten from the table as 1.96
The formula for the confidence interval for p is
![^(\prime)p-z_(\alpha I2)\sqrt[]{(^(\prime)p^(\prime)q)/(n)}<p>Substitute into the formula</p>[tex]\begin{gathered} ^(\prime)p-z_(\alpha I2)z_(\alpha I2)\sqrt[]{(^(\prime)p^(\prime)q)/(n)} \\ ^(\prime)p=0.122;^(\prime)q=0.878;z_(\alpha I2)=1.96 \\ =0.122-1.96_{}\sqrt[]{(0.122*0.878)/(450)} \\ =0.122-1.96\sqrt[]{(0.107116)/(450)} \\ =0.122-1.96\sqrt[]{0.0002380356} \\ =0.122-1.966(0.015428) \\ =0.122-0.03024 \\ =0.09176 \\ =0.092(3\text{ decimal places)} \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/exyn3htnshhz9w00jbiw.png)
![\begin{gathered} z_(\alpha I2)\sqrt[]{(^(\prime)p^(\prime)q)/(n)}\text{was calculated to be 0.03024} \\ \text{Therefore} \\ ^(\prime)p+z_(\alpha I2)\sqrt[]{(^(\prime)p^(\prime)q)/(n)}=0.122+0.03024=0.15224 \\ =0.152(3\text{decimal places)} \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/a0zwm8aliyuaegmppudc.png)
Hence, the confidence interval is 0.092