Answer:
The constant rate of acceleration required in order to accomplish this is 1.921 feet per square second.
Step-by-step explanation:
Let suppose that car accelerates uniformly in a rectilinear motion. Given that initial and final speeds and travelled distances are known, then the acceleration needed by the vehicle (
 ), measured in feet per square second, is determined by the following kinematic formula:
), measured in feet per square second, is determined by the following kinematic formula:
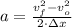 (1)
(1)
Where:
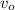 ,
,
 - Initial and final speeds, measured in feet per second.
- Initial and final speeds, measured in feet per second.
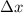 - Travelled distance, measured in feet.
- Travelled distance, measured in feet.
If we know that
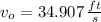 ,
,
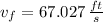 and
and
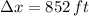 , then acceleration needed to accomplish the task is:
, then acceleration needed to accomplish the task is:
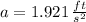
The constant rate of acceleration required in order to accomplish this is 1.921 feet per square second.