Question

The operator of a pumping station has observed that demand for water during early afternoon hours has an approximately exponential distribution with mean 100 cfs (cubic feet per second). (a) Find the probability that the demand will exceed 240 cfs during the early afternoon on a randomly selected day. (Round your answer to four decimal places.)

Answer 1

0.0907 = 9.07% probability that the demand will exceed 240 cfs during the early afternoon on a randomly selected day.

Explanation:

Exponential distribution:

The exponential probability distribution, with mean m, is described by the following equation:

`f(x) = \mu e^(-\mu x)`

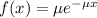
In which
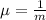`\mu = (1)/(m)` is the decay parameter.

The probability that x is lower or equal to a is given by:

`P(X \leq x) = \int\limits^a_0 {f(x)} \, dx`

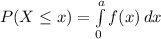
Which has the following solution:

`P(X \leq x) = 1 - e^(-\mu x)`

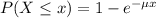
The probability of finding a value higher than x is:

`P(X > x) = 1 - P(X \leq x) = 1 - (1 - e^(-\mu x)) = e^(-\mu x)`

Exponential distribution with mean 100 cfs (cubic feet per second):

This means that
`m = 100, \mu = (1)/(100) = 0.01`

(a) Find the probability that the demand will exceed 240 cfs during the early afternoon on a randomly selected day.

`P(X > 240) = e^(-240*0.01) = 0.0907`

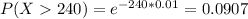
0.0907 = 9.07% probability that the demand will exceed 240 cfs during the early afternoon on a randomly selected day.